Up to this point, we have learned how to create graphs that reflect linear equations and how to construct linear equations given graphs. In this section, we will introduce two characteristics that pairs of lines can have so that we can continue to construct linear equations with various properties.
learn what it means for two lines to be parallel
learn what it means for two lines to be perpendicular
determine whether lines are parallel, perpendicular, or neither from their equations
construct linear equations for lines with various properties
The main topics of this section are also presented in the following videos:
Parallel lines are lines in the same plane that never intersect. Two different lines in the same plane are parallel if their slopes are the same; in symbols, if the slope of the first line is \(m_1\) and the slope of a different line is \(m_2\text{,}\) then the slopes are parallel if \(m_1=m_2\text{.}\)
Find an equation of the line passing through \((4,1)\) and parallel to \(x-2y=-2\text{.}\)
To find the slope of the given line, solve for \(y\text{.}\)
Here the given line has slope \(m_1=\frac{1}{2}\text{.}\) The line we are constructing is parallel to this line and will therefore have the same slope, so \(m_2=\frac{1}{2}\text{.}\) Since we are given a point and we now have the slope, we will choose to use point-slope form of a linear equations to determine the slope-intercept form of the equation.
Our point is \((4,1)\) and our slope is \(m=\frac{1}{2}\text{.}\)
The equation of the line is given by \(y-1 = \dfrac{1}{2}(x-4)\text{.}\)
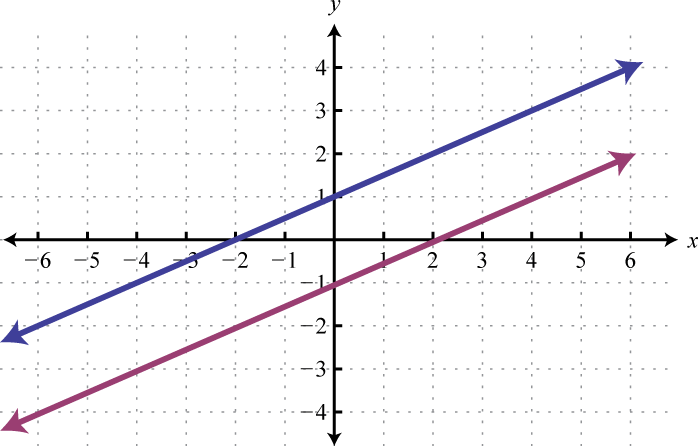
It is important to have a geometric understanding of this question. The line we were given, \(x-2y=-2\text{,}\) is shown in blue. The line we constructed, \(y-1=\frac{1}{2}(x-4)\text{,}\) is shown in magenta. notice that the slope is the same at the given line, but they are distinct lines (that is, they do not have the same \(y\)-intercept).
Perpendicular lines are lines in the same plane that intersect at right angles (90 degrees). Two nonvertical lines, in the same plane with slopes \(m_1\) and \(m_2\text{,}\) are perpendicular if the product of their slopes is \(-1\text{,}\) \(m_1\cdot m_2=-1\text{.}\) We can solve for \(m_1\) and obtain \(m_1=-\frac{1}{m_2}\text{.}\) In this form, we see that perpendicular lines have slopes that are opposite reciprocals. In general, given non-zero real numbers \(a\) and \(b\text{,}\) if the slope of the first line is given by \(m_1=\frac{a}{b}\text{,}\) then the slope of the perpendicular line is \(m_2=-\frac{b}{a}\text{.}\)
For example, the opposite reciprocal of \(m_1=-\frac{3}{5}\) is \(m_2=\frac{5}{3}\text{.}\) We can verify that two slopes produce perpendicular lines if their product is \(-1\text{.}\)
Find an equation of the line passing through \((-5,-2)\) and perpendicular to the graph of \(x+4y=4\text{.}\)
To find the slope of the given line, solve for \(y\text{.}\)
The given line has slope \(m_1=-\frac{1}{4}\text{.}\) Since we are constructing a line that is perpendicular to this line, their slopes should be opposite reciprocals, and thus the line we are constructing should have a slope of \(m_2=+\frac{4}{1}=4\text{.}\) Now we can substitute the slope, \(m_2=4\text{,}\) and the given point, \((-5,-2)\text{,}\) into point-slope form:
The equation of the perpendicular line is given by \(y+2=4(x+5)\text{.}\)
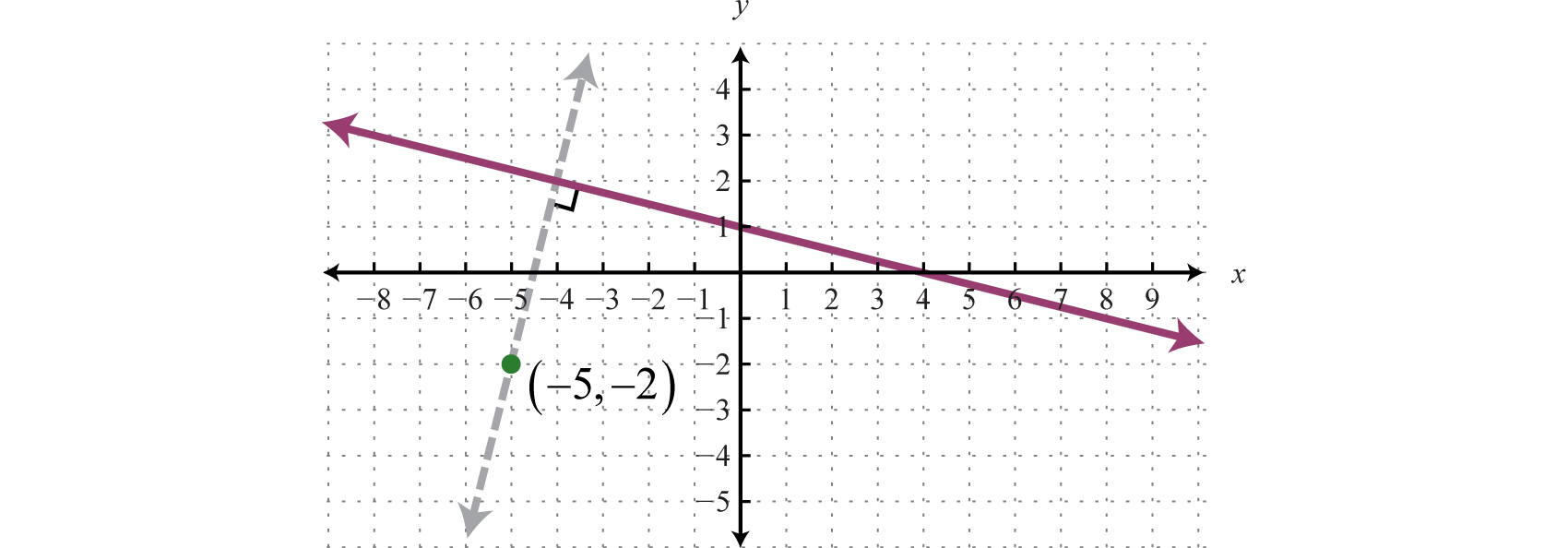
Geometrically, we see that the graph of \(y=4x+18\text{,}\) shown as the dashed line in the graph, passes through \((-5,-2)\) and is perpendicular to the graph of \(y=-\frac{1}{4}x+1\text{.}\)
Find an equation of the line passing through \((-5,-1)\) and perpendicular to \(\frac{1}{3}x-\frac{1}{2}y=-2\text{.}\)
To find the slope of the given line, we solve for \(y\text{:}\)
The given line has slope \(m_1=\frac{2}{3}\text{,}\) therefore the line we are constructing must have a slope of \(m_2=-\frac{3}{2}\text{.}\) Using this and the point \((-5,-1)\text{,}\) we can use point-slope form to write the following equation:
