Nathan Wakefield, Christine Kelley, Marla Williams, Michelle Haver, Lawrence Seminario-Romero, Robert Huben, Aurora Marks, Stephanie Prahl, Based upon Active Calculus by Matthew Boelkins
Section1.1Introduction to Continuity
Motivating Questions
What does it mean to say that a function \(f\) is continuous at \(x = a\text{?}\)
What does it mean to say that a function \(f\) is continuous everywhere?
Supplemental Videos
The main topics of this section are also presented in the following videos:
In this section we introduce the idea of a continuous function. Many of the results in calculus require that the functions be continuous, so having a strong understanding of continuous functions will be very important.
SubsectionBeing Continuous at a Point
Intuitively, a function is continuous if we can draw its graph without ever lifting our pencil from the page. Alternatively, we might say that the graph of a continuous function has no jumps or holes in it.
Figure1.1Functions \(f\text{,}\) \(g\text{,}\) and \(h\) that demonstrate subtly different behaviors at \(a = 1\text{.}\)
First consider the function in the left-most graph above. Note that \(f(1)\) is not defined, which leads to the resulting hole in the graph of \(f\) at \(a = 1\text{.}\) If you were to draw the graph of \(f\) yourself then you would need to lift your pencil when you reached \(f(1)\text{.}\) We will naturally say that \(f\) is not continuous at \(a = 1\). For the function \(g\text{,}\) we observe that while \(g(1)\) is defined, the value of \(g(1) = 2\) is not what you would expect. Specifically, you would expect \(g(1)\) to be 3, not 2. Thus, to draw the graph of \(g\) you would need to lift your pencil at \(a=1\text{.}\) Again, we will say that \(g\) is not continuous at \(a=1\text{,}\) even though the function is defined at \(a = 1\text{.}\) Finally, the function \(h\) appears to be the most well-behaved of all three, since at \(a = 1\) the function value is what you might expect it to be if you were to try and draw the graph of the function without lifting your pencil. In this case we would say that \(h\) is continuous at \(a=1\text{.}\)
The above examples demonstrate a discontinuity commonly know as a removable discontinuity. This is, however, not the only way in which a function can be discontinuous. Another type of discontinuity is the so-called jump discontinuity illustrated below.
Figure1.2Places where functions make a jump also produce discontinuities.
A third type of discontinuity is the so-called infinite discontinuity. Infinite discontinuities exist at points where the values of a function diverge to infinity. A classic example of an infinite discontinuity is the point \(x=0\) for the function \(f(x)=\frac{1}{x}\text{;}\) you can see the behavior of the infinite discontinuity in the graph of \(y=f(x)\) below.
Figure1.3A plot of the function \(y=f(x)\) for the function \(f(x)=\frac{1}{x}\text{.}\)
We first consider a graph of \(y=f(x)\text{,}\) shown below.
Figure1.6A plot of the function \(y=f(x)\text{.}\)
Visual inspection of the graph certainly indicates discontinuities. However, we can make our visual inspection more precise through a little algebra. We start by expanding the numerator and denominator of the function. Specifically, we have
From here we see that there are two \(x\)-values at which the function is undefined: at \(x=-4,4\text{.}\) Since the term \(x+4\) occurs in both the numerator and denominator, the point \(x=-4\) is a removable discontinuity. Since the term \(x-4\) occurs only in the denominator, \(x=4\) is an infinite discontinuity.
Note1.7
Rational functions can be a challenging topic. For a more complete discussion of rational functions we refer the reader to Short-Run Behavior of Rational Functions
SubsectionPiecewise Functions
In many cases a simple function like \(f(x)=x^2\) may not fully describe the behavior of a phenomenon. In some of these cases we can turn to piecewise functions to give us the tools we need.
Piecewise Function
A piecewise-defined function is one which is defined not by a single equation, but by two or more. Each equation is valid for some interval.
Think of the plane as divided into two regions by the vertical line \(x = 1\text{,}\) as shown in Figure1.9. In the left-hand region (\(x \le 1\)), we graph the line \(y = x + 1\text{.}\) (The fastest way to graph the line is to plot its intercepts, \((-1, 0)\) and \((0, 1)\text{.}\))
Notice that the value \(x = 1\) is included in the first region, so \(f (1) = 1 + 1 = 2\text{,}\) and the point \((1, 2)\) is included on the graph. We indicate this with a solid dot at the point \((1, 2)\text{.}\)
In the right-hand region (\(x \gt 1\)), we graph the horizontal line \(y = 3\text{.}\) The value \(x = 1\) is not included in the second region, so the point \((1, 3)\) is not part of the graph. We indicate this with an open circle at the point \((1, 3)\text{.}\)
Figure1.10
The jumps that a piecewise function possesses make piecewise functions a natural place in which to explore continuity.
Example1.11
The function
\begin{equation*}
h(x)=\begin{cases} 4x^2 \amp \text{ if } x\leq 1 \\ 3x+3 \amp \text{ if } x\gt1 \end{cases}
\end{equation*}
is discontinuous at \(x=1\text{.}\) It is continuous on any interval that does not include \(x=1\text{.}\)
Not only can we ask questions about when a piecewise function is continuous, we can also ask questions about how to make a piecewise function continuous by varying parameters.
Example1.12
Consider the piecewise function
\begin{equation*}
D(x)=\begin{cases}
4x^2-k\amp\text{ if } x \lt 2,\\
kx+1\amp\text{ if } x \geq 2.\\
\end{cases}
\end{equation*}
Find the value of \(k\) to make this function continuous for all \(x\text{.}\)
The point where the function may not be continuous is at the potential jump-point. Specifically, when \(x=2\text{.}\) For the function to be continuous we need the two branches of the function to meet when \(x=2\text{.}\) That is we need \(4\cdot(2)^2-k=k\cdot(2)+1\) which is equivalent to \(k=5\text{.}\) The figure below shows a graph of \(y=D(x)\) for \(k=5\text{.}\)
Figure1.13A plot of the function \(y=D(x)\text{,}\) with the parameter \(k=5\text{.}\)
SubsectionThe Intermediate Value Theorem
Up to this point we have talked about the continuity of a function at a point. Before stating the Intermediate Value Theorem we begin by defining a continuous function. We will formalize this definition in Section1.2 with the introduction of limits.
Continuous Function
A function is said to be continuous on an interval \((a,b)\) if the function has no points of discontinuity on that interval.
One of the most important theorems in Calculus is the Intermediate Value Theorem, which we state formally below.
Intermediate Value Theorem
Suppose \(f\) is continuous on a closed interval \([a,b]\text{.}\) If \(k\) is any number between \(f(a)\) and \(f(b)\text{,}\) then there is at least one number \(c\) in \([a,b]\) such that \(f(c)=k\text{.}\)
The figure below illustrates the theorem. The function \(f(x) \text{,}\) whose graph is drawn in black, is continuous on the closed interval \([a,b] \text{.}\) Suppose we choose a number \(k \) between \(f(a) \) and \(f(b) \text{.}\) The Intermediate Value Theorem says that the horizontal line \(y = k \) must intersect the graph of \(f(x) \) in at least one point.
Figure1.15The Intermediate Value Theorem in action.
In the figure, the line \(y = k \) intersects the graph of \(f(x) \) three times. The \(x \)-coordinate of any one of these points of intersection is a number \(c \) such that \(f(c) = k \text{.}\)
Example1.16
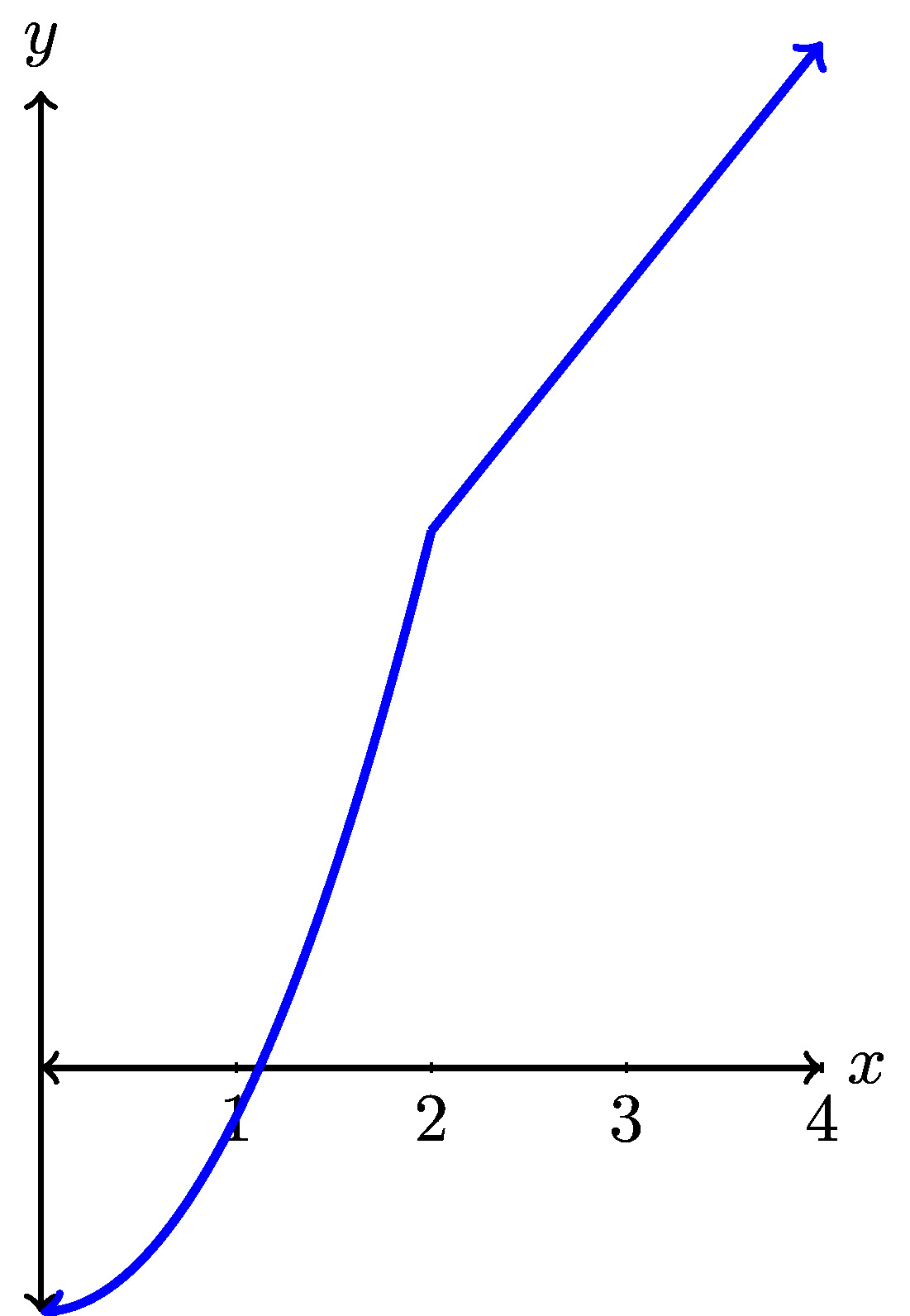
Amber measures herself before going to bed and is 67 inches tall. She measures herself again when she wakes up 8 hours later, and is now 68 inches tall. Let \(h(t)\) be Amber's height in inches \(t\) hours after the first measurement. Then \(h(0)=67\) and \(h(8)=68\text{.}\)
Do you think there is a time between when Amber goes to bed and when she wakes up that she is 67.5 inches tall?
Since Amber is likely growing continuously (i.e., she did not jump from 67 inches to 68 inches instantly), the Intermediate Value Theorem holds for the height function \(h(t)\text{.}\) The Intermediate Value Theorem states that at some time while Amber slept, she measured 67.5 inches tall.
Example1.17
Consider the function \(f(x)=x^3-3x^2+3x-4\text{.}\) Use the Intermediate Value Theorem to show that \(f(x)\) has a root between \(x=2\) and\(x=3\text{.}\)
We want to show that there is some number \(c\) between 2 and 3 such that \(f(c)=0\text{.}\) We consider the value of the function \(f\) at \(x=2\) and \(x=3\text{:}\)
Thus \(f(2)\lt 0\lt f(3)\text{;}\) in other words, 0 is a number between \(f(2)\) and \(f(3)\text{.}\) The function \(f\) is a polynomial, so it is a continuous function. Thus the Intermediate Value Theorem says there is a number \(c\) between 2 and 3 such that \(f(c)=0\text{.}\) In other words, \(f\) has a root \(c\) in the interval \((2,3)\text{.}\)
