The Curl of a Vector Field

Section 4.5 The Curl of a Vector Field

Motivating Questions

What is meant by rotation of a vector field in a plane?
How can a two-dimensional measurement of rotation be generalized to work in three dimensions?
How can the rotational strength of a vector field be measured?

In many physical settings, it is useful to measure the rotational strength of a vector field at a local scale. For instance, the vector field on the left of Figure 4.5.1 shows a vector field which flows in a counterclockwise fashion around the origin. The vector field on the right of Figure 4.5.1 shows a vector field which does not have a rotational aspect to its flow.

described in detail following the image — 🔗
Figure 4.5.1. Examples of vector fields with global rotation properties (left) and without rotation in either the global or local sense (right)

🔗

These global ideas of rotation are nice, but are not always visually apparent or may only appear in some regions. For instance, the vector field on the left of Figure 4.5.2 seems to have rotation around at least a couple of different points. In each quadrant, the vector field on the right of Figure 4.5.2 has different rotational patterns.

🔗

Preview Activity 4.5.1.

🔗

We would like to understand and measure rotation of a vector field near a particular point. In order to investigate this concept, we will look at some two-dimensional vector fields and think about whether the vector field shown will rotate a small pinwheel or spinner placed at a particular location. The sort of spinner we imagine is illustrated in Figure 4.5.3. It consists of a central axis with a four-bladed paddle placed at one end of the axis. We imagine that the spinner is anchored at a point and the vector field, perhaps thought of as a fluid flow or wind velocity vector field, pushes against the blades of the spinner’s paddle.

🔗

(a)

🔗

To begin our investigation of the rotation of a spinner in a vector field, we will look at the vector field

F

in Figure 4.5.4. As you think about these questions, draw an “X” at each of the points about which you are asked and consider the vector field as being the pattern of a wind blowing across the plane.

🔗

(i)

🔗

Draw a vector on the top right blade of your spinner that represents how the wind will push on that blade. Next, draw a vector on each of the other blades of your spinner that represents how the wind will push on that blade.

🔗

(ii)

🔗

Use your vector representations from the previous part to describe if a small spinner placed at the origin would spin clockwise, counterclockwise, or not spin at all.

🔗

(iii)

🔗

Now we would like to look at the rotational strength of

F

at the point

(1, 1) .

As before, draw a spinner at this point and draw vectors on each of the blades to represent how the wind will push on that blade. It is important at this stage to draw the relative lengths of the vectors on each blade to scale so you can see which blades have a larger force due to the wind.

🔗

(iv)

🔗

Use your vector representations from the previous part to describe if a small spinner placed at at

(1, 1)

would spin clockwise, counterclockwise, or not spin at all. You should pay attention to which blades will have a stronger force (in comparison to the blade on the other side).

🔗

(v)

🔗

Would a small spinner placed at

(- 2, 1)

spin clockwise, counterclockwise, or not spin at all? Draw a representation of a spinner if necessary to demonstrate your ideas.

🔗

(vi)

🔗

Are there any points on the

x y

-plane where the spinner would not turn counterclockwise?

🔗

(b)

🔗

For this part, look at the vector field

G

in Figure 4.5.5.

🔗

(i)

🔗

Would a small spinner placed at the origin spin clockwise, counterclockwise, or not spin at all?

🔗

(ii)

🔗

Would a small spinner placed at

(1, 1)

spin clockwise, counterclockwise, or not spin at all?

🔗

(iii)

🔗

Would a small spinner placed at

(- 2, 1)

spin clockwise, counterclockwise, or not spin at all?

🔗

(iv)

🔗

Are there any points in the

x y

-plane where the spinner would turn differently than your descriptions in the previous part of this problem?

🔗

(c)

🔗

For this part, we consider the vector field

H

shown in Figure 4.5.6.

🔗

(i)

🔗

Would a small spinner placed at the origin spin clockwise, counterclockwise, or not spin at all?

🔗

(ii)

🔗

Would a small spinner placed at

(1, 1)

spin clockwise, counterclockwise, or not spin at all?

🔗

(iii)

🔗

Would a small spinner placed at

(- 2, 1)

spin clockwise, counterclockwise, or not spin at all?

🔗

(iv)

🔗

Are there any points on the

x y

-plane where the spinner would turn differently than your descriptions in the previous part of this problem?

🔗

(d)

🔗

For this part, look at the vector field in Figure 4.5.7. You may find it useful to recenter the plot on your point by changing

x_{0}

and

y_{0} .

You may also zoom in on your point by using the slider labeled “zoom”.

Figure 4.5.7. A SageMath Interact to examine vector fields

🔗

(i)

🔗

Would a small spinner placed at the origin spin clockwise, counterclockwise, or not spin at all?

🔗

(ii)

🔗

Would a small spinner placed at

(0, 2)

spin clockwise, counterclockwise, or not spin at all?

🔗

(iii)

🔗

Would a small spinner placed at

(- 2, 1)

spin clockwise, counterclockwise, or not spin at all?

🔗

(iv)

🔗

For each of the following spinner behaviors, give a point in the

x y

-plane (different than the ones used in the previous parts) where a spinner would:

Turn clockwise.
Turn counterclockwise.
Not turn at all.

🔗

Subsection 4.5.1 Measuring the Circulation Density of Vector Field in $R^{2}$

🔗

In this subsection, we will develop the measurement of the circulation density for a two-dimensional vector field. We will use this measurement to generalize to a notion of rotational strength in higher dimensional cases in the next subsection.

🔗

We will start by measuring the circulation of a vector field on a path around the point

(a, b)

and use this measurement to define circulation density. Specifically, we will measure the circulation of a vector field as we move around a square centered at

(a, b) .

Using this measurement, we will calculate the circulation density by dividing our measurement by the area enclosed. This will allow us to compare our measurement across regions of different sizes. By taking the limit of this circulation density as the square’s side length goes to zero, we will have the circulation density at the point

(a, b) .

Just as in our discussion of Subsection 4.9.1, we will look at a two dimensional setting first, then examine how our argument can be generalized to higher dimensions.

🔗

Let’s start by measuring the circulation around a square with side length

2 h

centered at a point

(a, b) .

Namely, we will look at the line integral of our vector field as we move along the square curve shown in Figure 4.5.8.

🔗

We can parametrize the top edge of the square using the parametrization

r_{top} (t) = ⟨ a - t, b + h ⟩

🔗

for

- h \leq t \leq h .

Similarly, the bottom, right, and left can be parametrized (over the same range of values for

t

) as follows:

\begin{aligned} r_{bottom} (t) & = ⟨ a + t, b - h ⟩ \\ r_{right} (t) & = ⟨ a + h, b + t ⟩ \\ r_{left} (t) & = ⟨ a - h, b - t ⟩ \end{aligned}

🔗

In order to measure the circulation, we want to know how much of the vector field

F = ⟨ F_{1}, F_{2} ⟩

is parallel to the direction of travel. Thus, along each of the sides of the box, we only need to look at one of the components of the vector field. The only contribution to the circulation on the top and bottom comes from the horizontal component

F_{1} .

Similarly, the vertical component

F_{2}

is all that contributes on the right and left sides. This means that we can simplify our line integrals considerably once we apply Theorem 4.3.2. For instance, the circulation on the top can be written as

\int_{top} F \cdot d r = \int_{- h}^{h} ⟨ F_{1}, F_{2} ⟩ \cdot ⟨ - 1, 0 ⟩ d t = \int_{- h}^{h} - F_{1} (a - t, b + h) d t

🔗

Similarly, we can simplify the appropriate line integrals on the other sides (ordered below as top, left, bottom, right) to get the total circulation around the square to be

\begin{aligned} Total Circulation = & \int_{Square} F \cdot d r \\ = & \int_{- h}^{h} - F_{1} (a - t, b + h) d t + \int_{- h}^{h} - F_{2} (a - h, b - t) d t \\ + \int_{- h}^{h} F_{1} (a + t, b - h) d t + \int_{- h}^{h} F_{2} (a + h, b + t) d t \end{aligned}

🔗

Note that the sign on the top and the left sides ends up being switched because the direction of travel on these sides is in the negative coordinate direction. It is important to note that by convention, we talk about “positive” rotation being counterclockwise and “negative” rotation being clockwise when looking at these planar graphs. This will be discussed more fully in Subsection 4.5.2.

🔗

As with our development of divergence, we apply the Mean Value Theorem to the four integrals above to simplify future calculations. Specifically, the Mean Value Theorem applied to the first integral tells us that there is a value to get a value

a_{top}^{*}

in the interval

(a - h, a + h)

such that

\int_{- h}^{h} - F_{1} (a - t, b + h) d t = - 2 h (F_{1} (a_{top}^{*}, b + h))

🔗

Applying the same arguments to the other three integrals in our Total Circulation calculation gives

\begin{aligned} Total Circulation = & \int_{Square} F \cdot d r \\ = & \int_{- h}^{h} - F_{1} (a - t, b + h) d t + \int_{- h}^{h} - F_{2} (a - h, b - t) d t \\ + \int_{- h}^{h} F_{1} (a + t, b - h) d t + \int_{- h}^{h} F_{2} (a + h, b + t) d t \\ = & - 2 h F_{1} (a_{top}^{*}, b + h) - 2 h F_{2} (a - h, b_{left}^{*}) \\ + 2 h F_{1} (a_{bottom}^{*}, b - h) + 2 h F_{2} (a + h, b_{right}^{*}) \end{aligned}

🔗

Here we have

a_{top}^{*}, a_{bottom}^{*} \in (a - h, a + h)

and

b_{left}^{*}, b_{right}^{*} \in (b - h, b + h) .

We can regroup these terms to get the following expression for the total circulation around our square:

2 h (F_{2} (a + h, b_{right}^{*}) - F_{2} (a - h, b_{left}^{*})) - 2 h (F_{1} (a_{top}^{*}, b + h) - F_{1} (a_{bottom}^{*}, b - h))

🔗

A larger square is likely to have a larger total circulation since there is more distance to accumulate how much the vector field is moving in the same direction as our path. This is noticeable in the formula above for total circulation, as the side length

2 h

of the square appears in the formula. In order to compare our rotational or circulation ideas over different sizes of squares, we will now look at the circulation density (strength of rotation per unit area), which can be computed directly from our total circulation measurement. We can take the limit of the circulation density as we shrink the square to the central point

(a, b)

and get a measurement rotational strength of our vector field at a point.

🔗

We will compute our circulation density as

Circulation Density = lim_{h \to 0} \frac{Total Circulation}{Area of Square} .

🔗

Since the area of the square is

(2 h)^{2} = 4 h^{2},

this gives us that the circulation density is

lim_{h \to 0} \frac{F_{2} (a + h, b_{right}^{*}) - F_{2} (a - h, b_{left}^{*})}{2 h} - \frac{F_{1} (a_{top}^{*}, b + h) - F_{1} (a_{bottom}^{*}, b - h)}{2 h} .

🔗

Recall the central difference method of estimating derivatives from Section 1.5.2

activecalculus.org/single/sec-1-5-units.html#VjW

and notice that as

h \to 0,

a_{top}^{*}, a_{bottom}^{*}

must go to

a

and

b_{left}^{*}, b_{right}^{*}

must go to

b .

Therefore, our circulation density measurement at a point is

Circulation Density at the point (a, b) = \frac{\partial F_{2}}{\partial x} (a, b) - \frac{\partial F_{1}}{\partial y} (a, b)

🔗

You may have noticed that this argument had a similar flow to the development of divergence in Section 4.9. However, the result here is different because the property of the vector field we were trying to measure was different. Because divergence was developed to measure the change in the strength of the vector field (without regard to the direction), divergence is computed using the partial derivatives of the horizontal component of the vector field with respect to the horizontal variable and the vertical component of the vector field with respect to the vertical variable. The circulation density we just developed measures how the direction of the vector field is changing, and thus uses partial derivatives of the components with respect to the transverse variable. In other words, the rotational aspects of the vector field depend on how the horizontal component of the vector field changes when we move vertically (and vice versa).

🔗

We could have used shapes other than a square in the development of the circulation density, but the square allows for calculations that are easier to understand as the area of the shape decreases.

🔗

Activity 4.5.2. Matching Visual Measurements to Algebraic Calculations.

🔗

Remember that the circulation density of a vector field

F = ⟨ F_{1}, F_{2} ⟩

at a point

(a, b)

is calculated as

Circulation Density at the point (a, b) = \frac{\partial F_{2}}{\partial x} (a, b) - \frac{\partial F_{1}}{\partial y} (a, b) .

🔗

The circulation density will be positive when a small spinner placed at

(a, b)

will spin counterclockwise, negative when the spinner will move clockwise, and zero when the spinner does not rotate.

🔗

For each of the vector fields given below, answer the following questions:

What is the formula for the circulation density of the vector field?
For what points will you have a positive circulation density?
For what points will you have a negative circulation density?
For what points will you have a zero circulation density?
Are your answers to the above questions consistent with what you found in a part of Preview Activity 4.5.1? If so, which part and why?

🔗

(a)

🔗

⟨ - y, x ⟩

🔗

(b)

🔗

⟨ x, y ⟩

🔗

(c)

🔗

⟨ 1 - x, x y ⟩

🔗

Subsection 4.5.2 Measuring Rotation in Three Dimensions

🔗

The previous subsection showed how we can measure the circulation density, or strength of rotation, at a point for a two-dimensional vector field. In this subsection, we will look at how this two-dimensional measurement can be used to define circulation density for three dimensional vector fields.

🔗

Figure 4.5.9. Rotation as a vector on the

x y

-plane

🔗

We first need to consider how we want to represent and measure rotation in three dimensions. When we developed the circulation density on the

x y

-plane, we measured the rotational strength at a point

(a, b)

with an axis of rotation coming out of the

x y

-plane. This corresponds to the axis of rotation being given by the blue vector in Figure 4.5.9. Remember that “positive” rotation corresponds to counterclockwise rotation in the

x y

-plane. We can generalize this idea to think of rotation in three dimensions as being represented by a vector where

the direction of the vector represents the axis of rotation and
the magnitude of the vector represents the strength of the rotation.

🔗

By convention, we consider positive “rotation” to correspond to counterclockwise rotation when the vector field is viewed looking from the terminal point of the vector to its initial point. In Figure 4.5.10, you can see that each vector corresponds to the rotation displayed and is consistent with the conventions described above.

🔗

Figure 4.5.10. Rotation as a vector on each coordinate plane

🔗

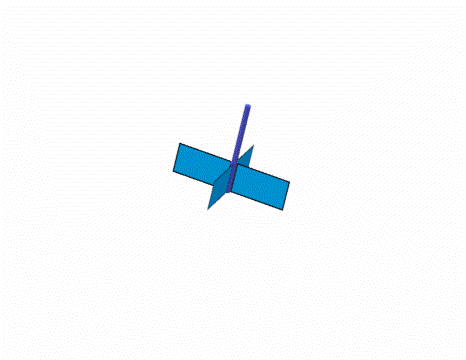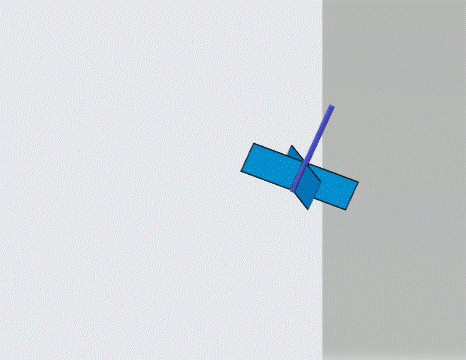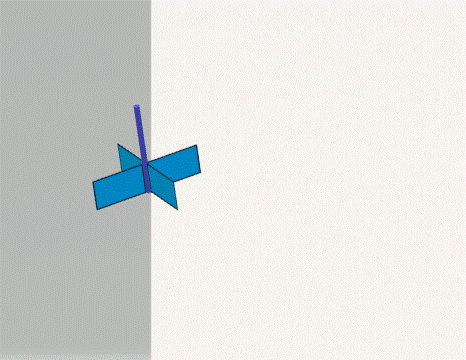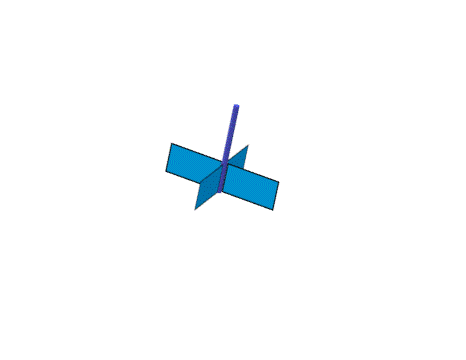
If you look carefully at Figure 4.5.10, you can see that the vector shown in blue will be parallel to

k

and will represent rotations on planes of the form

z = c .

Similarly, the vector shown in yellow will be parallel to

i

and will represent rotations on planes of the form

x = a .

When looking down the blue and yellow vectors (from the terminal to the initial point), you can see the positive coordinate axes as pointing to the right and up

When placing the

x

-axis horizontally for the blue vector and placing the

y

-axis horizontally for the yellow vector

(as we would expect on a two-dimensional plot). In contrast, when you look down the magenta vector (from the terminal to the initial point), the positive coordinate axes (the

x

and

z

axes) point to the left and up.

When placing the

x

-axis horizontally.

In fact, when we look down the purple vector the

x z

-plane is flipped. A positive rotation on a plane of the form

y = b

will correspond to a rotation vector that is in the direction of

- j .

This is a consequence of the right-handed coordinate system and our right-handed idea of rotation, as reflected in the relationships amongst the vectors

i, j v k

recalled below:

i \times j = k i \times k = - j j \times k = i

🔗

With this idea of using a vector to represent the rotation of some element, we have enabled all of the tools that vectors allow, especially the ideas of projection and linear combinations. Remember that the projection of a vector

v

onto a vector

u

will give the vector component of

v

that is parallel to

u .

In other words, the projection of

v

onto

u

is how much of

v

is parallel to

u .

The projection formula will allow us to take a vector representation of rotation and determine how much rotation happens around a given axis of rotation.

🔗

Recall that we have used a set of coordinate vectors (namely

i,

j,

and

k

) to write other vectors as a linear combination of our base vectors. In other words, a vector

⟨ a, b, c ⟩

R^{3}

can be written in the form

a i + b j + c k .

🔗

Figure 4.5.11. The black vector,

⟨ a, b, c ⟩,

is decomposed into parts parallel to

i,

j,

and

k .

🔗

The linear combination of vectors will allow us to build the total rotation vector by measuring the rotational strength in the direction of three coordinate direction vectors. Just as in Figure 4.5.11, we will build our total rotation vector as a sum of

i,

j,

and

k .

A rotation vector in the direction of

k

at a point

(a, b, c)

will measure the circulation density at our point restricted to the trace plane

z = c .

Similarly the rotation vectors in the directions

i

and

j

will measure the circulation density on the trace planes

x = a

and

y = b,

respectively.

🔗

Subsection 4.5.3 Circulation Density in Three Dimensions

🔗

The previous subsection discussed how we can view the amount of rotation of a three-dimensional vector field in a trace plane parallel to the

x y

x z

-, or

y z

-plane and recalled that vectors in

R^{3}

can be written as a linear combination of the vectors

i,

j,

and

k .

We use our definition of curl for a two-dimensional vector field to measure the amount of rotation in the appropriate trace planes, which leads to the following definition.

🔗

Definition 4.5.12.

🔗

Let

F = ⟨ F_{1}, F_{2}, F_{3} ⟩

be a three-dimensional vector field. The curl of

F

is given by

curl (F) = (\frac{\partial F_{3}}{\partial y} - \frac{\partial F_{2}}{\partial z}) i - (\frac{\partial F_{3}}{\partial x} - \frac{\partial F_{1}}{\partial z}) j + (\frac{\partial F_{2}}{\partial x} - \frac{\partial F_{1}}{\partial y}) k

🔗

The curl is exactly the rotational description described at the end of Subsection 4.5.2. When evaluated at a point

(a, b, c),

the first component of the curl will measure the circulation density of the vector field restricted to the plane

x = a .

Similarly, the second and third components of the curl will measure the circulation density of the vector field restricted to the planes

y = b

and

z = c,

respectively.

🔗

Activity 4.5.3. Estimating Curl in Three Dimensions.

🔗

(a)

🔗

Look at the vector field

F

plotted in Figure 4.5.13. You can adjust the size of the region around

(1, 1, - 2)

over which the vector field is plotted using the “Zoom” slider. The “Density” slider allows you to adjust the number of vectors plotted. Can you see any rotation in the three dimensional vector field plot at the point

(1, 1, - 2) ?

Can you tell how a spinner placed at

(1, 1, - 2)

would rotate? Along which axis? Try to state your answer as a vector representing the rotational strength of the vector field at

(1, 1, - 2) .

Figure 4.5.13. A vector field plotted in a region around the point

(1, 1, - 2)

🔗

(b)

🔗

You likely found it difficult to decide how you thought a spinner might rotate in this new, three-dimensional setting. Let’s look at the vector field in the plane

z = - 2,

as displayed in Figure 4.5.14. Do you think a spinner placed on the red point would rotate clockwise, counterclockwise, or not rotate? If the spinner will rotate, you should think about what the axis of rotation would be and whether the rotation should be positive or negative. Summarize your result as a vector representing the rotational strength of

F

in the plane

z = - 2 .

🔗

(c)

🔗

Next we will look at the vector field in the plane

y = 1,

as displayed in Figure 4.5.15. Do you think a spinner placed on the red point would rotate clockwise, counterclockwise, or not rotate? If the spinner will rotate, you should think about what the axis of rotation would be and whether the rotation should be positive or negative. Summarize your result as a vector representing the rotational strength of your vector field in the plane

y = 1 .

Do you think the rotation in this figure is stronger or weaker than in Figure 4.5.14?

🔗

(d)

🔗

Finally, consider the trace of

F

in the plane

x = 1,

as displayed in Figure 4.5.16. Do you think a spinner placed on the red point would rotate clockwise, counterclockwise, or not rotate? If the spinner will rotate, you should think about what the axis of rotation would be and whether the rotation should be positive or negative. Summarize your result as a vector representing the rotational strength of your vector field in the plane

x = 1 .

🔗

(e)

🔗

Summarize your prediction to what you think the three-dimensional rotational strength of the vector field will be at the point

(1, 1, - 2)

in the form of three-dimensional vector.

Hint.

Add your results from the previous three steps.

🔗

(f)

🔗

Compute the curl of

F = ⟨ x - y, y + 2 z, x^{2} ⟩ .

Specifically, what is

curl (F)

at the point

(1, 1, - 2) ?

🔗

(g)

🔗

Compare the result of the curl calculation in part f to your prediction from part e. You likely found it difficult to estimate the magnitude, so your answer there may be incorrect. Hopefully, you did get the signs of the components and their relative strengths (i.e., which is biggest) correct. If you did not, go back and review the previous parts and explain why the calculated components match with the rotational strength for each of the three figures.

🔗

Subsection 4.5.4 Interpretation and Usage of Curl

🔗

The curl of a vector field is itself a vector field in that evaluating

curl (F)

at a point gives a vector. As we saw earlier in this section, the vector output of

curl (F)

represents the rotational strength of the vector field

F

as a linear combination of rotational strengths (or circulation densities) from two-dimensional planar descriptions. From our description of vectors as a representation of rotations, We can think of rotation in three dimensions as being represented by a vector where

the direction of the vector represents the axis of rotation and
the magnitude of the vector represents the strength of the rotation.

🔗

By convention, we consider positive “rotation” to correspond to counterclockwise rotation when the vector field is viewed looking from the end of the vector to the base.

🔗

With these conventions, the output vector of the curl evaluated at a point

P,

written as

curl (F) (P),

will have the following properties:

The direction of $curl (F) (P)$ will be the axis of rotation that has the strongest positive rotational strength.
The magnitude of $curl (F) (P)$ will be the circulation density of the vector field in the plane through $P$ with normal vector $curl (F) (P) .$

🔗

The following theorem allows us to use

curl (F)

to measure the rotational strength of

F

to around an arbitrary axis. This will be particularly useful to us in later sections.

🔗

Theorem 4.5.17.

🔗

The rotational strength of a vector field

F

around an axis given by a unit vector

v

at a point

P = (a, b, c)

can be computed by

(curl (F) (a, b, c)) \cdot v .

Proof.

This follows from the formula for the component of

curl (F) (a, b, c)

along

v

and the fact that

v

was selected to be a unit vector.

🔗

Activity 4.5.4.

🔗

In this activity, we will work on calculating curl algebraically and interpreting it.

🔗

(a)

🔗

Consider the vector field

F = ⟨ x, y, z ⟩ .

If we plot this vector field in any plane through the origin, we will see the vector field shown in Figure 4.5.18. This two-dimensional vector field has no rotation. The projection of

curl (F) (0, 0, 0)

onto any direction therefore must give the zero vector. The only vector that has a zero projection in every direction is the zero vector. Verify this geometric argument for the curl of

F

by doing the calculations necessary to show that

curl (⟨ x, y, z ⟩) = 0 .

🔗

(b)

🔗

Now consider the vector field

F = ⟨ - y, x, 0 ⟩,

which is shown in Figure 4.5.19. The figure includes both an interactive three-dimensional plot of

F

as well as a two-dimensional plot of

F

in the yellow plane, which can be adjusted using the “Angle of Plane” slider.

Figure 4.5.19. Two views of the vector field

⟨ - y, x, 0 ⟩

🔗

(i)

🔗

Based on the figure, would you estimate

curl (F)

is positive, negative, or zero at the origin? Does your answer change if you pick a different point?

🔗

(ii)

🔗

Calculate

curl (F)

algebraically. Does the curl of this vector field vary depending on the point at which the curl is measured?

🔗

(iii)

🔗

Explain why the rotational strength of the vector field on a titled plane through the origin will be given by

2 \cos (θ),

where

θ

is the angle between

k

and the normal vector of the tilted plane.

🔗

(c)

🔗

In Figure 4.5.20 you can plot a vector field in a region around a point of your choosing in order to look at the rotational properties of the vector field. The check box in Figure 4.5.20 will show the curl vector at the base point specified so you can make sense of your vector field and its curl.

Figure 4.5.20. A plot of the vector field

⟨ F_{1}, F_{2}, F_{3} ⟩

🔗

Use the figure to estimate the direction of

curl (⟨ x - z, x^{2} + z, x + \sin (y) ⟩)

at the point

(1, 2, - 1) .

Confirm your estimate by calculating the curl at this point algebraically. Is there any point at which the direction of greatest rotational strength of this vector field has negative

x

-component? If there is, find such a point. If not, explain why not.

🔗

Subsection 4.5.5 Summary

🔗

The circulation of a vector field on a closed path measures how strong the vector field moves in the direction of travel for the path. The circulation density of a two-dimensional vector field $F = ⟨ F_{1}, F_{2} ⟩$ is given by

$Circulation Density at the point (a, b) = \frac{\partial F_{2}}{\partial x} (a, b) - \frac{\partial F_{1}}{\partial y} (a, b) .$

The circulation density can be visualized in terms of how fast a very small spinner anchored to $(a, b)$ will rotate as a result of the force of $F .$
By considering the circulation density of a three-dimensional vector field $F = ⟨ F_{1}, F_{2}, F_{2} ⟩$ in planes parallel to the coordinate planes, we can calculate the curl of $F$ as

$curl (F) = (\frac{\partial F_{3}}{\partial y} - \frac{\partial F_{2}}{\partial z}) i - (\frac{\partial F_{3}}{\partial x} - \frac{\partial F_{1}}{\partial z}) j + (\frac{\partial F_{2}}{\partial x} - \frac{\partial F_{1}}{\partial y}) k .$
For a three-dimensional vector field $F,$ the vector $curl (F) (a, b, c)$ points in the direction of greatest rotational strength at the point $(a, b, c) .$ The magnitude of this vector measures the strength of the rotation. The strength of rotation around an axis determined by a unit vector $v$ is found by calculating $(curl (F) (a, b, c)) \cdot v$

Prev Top Next

Section 4.5 The Curl of a Vector Field

Motivating Questions

Preview Activity 4.5.1.

(a)

(i)

(ii)

(iii)

(iv)

(v)

(vi)

(b)

(i)

(ii)

(iii)

(iv)

(c)

(i)

(ii)

(iii)

(iv)

(d)

(i)

(ii)

(iii)

(iv)

Subsection 4.5.1 Measuring the Circulation Density of Vector Field in R2

Activity 4.5.2. Matching Visual Measurements to Algebraic Calculations.

(a)

(b)

(c)

Subsection 4.5.2 Measuring Rotation in Three Dimensions

Subsection 4.5.3 Circulation Density in Three Dimensions

Definition 4.5.12.

Activity 4.5.3. Estimating Curl in Three Dimensions.

(a)

(b)

(c)

(d)

(e)

(f)

(g)

Subsection 4.5.4 Interpretation and Usage of Curl

Theorem 4.5.17.

Proof.

Activity 4.5.4.

(a)

(b)

(i)

(ii)

(iii)

(c)

Subsection 4.5.5 Summary

Subsection 4.5.1 Measuring the Circulation Density of Vector Field in $R^{2}$